Using a simple example, we will show how superposition and entanglement can be used to obtain a better bomb detector than is classically possible. To do this, you will need to construct an optical circuit consisting of a photon source, two semi-permeable plates, two mirrors and two detectors.
A single photon, after passing through the first semi-permeable plate, is in superposition, meaning that it is simultaneously in the upper and lower paths. It then interferes with itself, resulting in quenching on the path to detector A and amplification on the path to detector B. Since there is destructive interference on the path to detector A, the probability of reading the photon at detector B is 100%. This is a kind of transformation of the two-slit experiment, in which the particle also interfered with itself, making it possible to create an interference pattern on the screen.
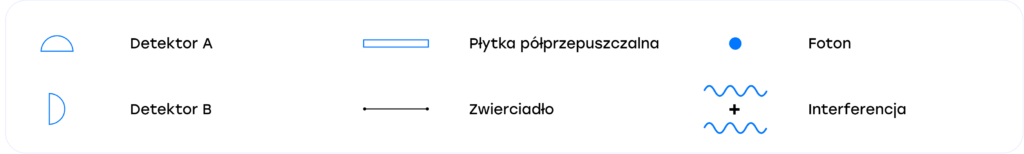
To the circuit on one of the photon paths, we will now add a bomb that explodes on contact with the photon. Since the bomb explosion is a classical act of measurement, there is a 50% probability that the bomb will explode. However, if the photon flew through the upper part of the circuit, it will again split into an upper and lower beam with a 50% probability, and therefore activate detectors A and B with equal probability.
A probability of 25% does not seem high, especially if the detonation of the bomb depends on it. However, it is still more than we would be able to do using the principles of classical mechanics, where it would be impossible to detect a bomb without detonating it. Besides, using other types of semi-permeable plates with transmission and reflection coefficients other than 50%, it is possible to get arbitrarily close to a probability of 100%.
Wykorzystamy bramki Hadamarda 
, w celu uzyskania superpozycji stanów, a także kontrolowaną bramkę 
lub 
, w celu splątania ze sobą zmiennych reprezentujących foton i bombę. Pomiar wyjścia obwodu będzie teraz procesem probabilistycznym. Interesujący nas wynik, w którym bomba nie wybuchła i aktywowany został detektor A, powinniśmy odczytać z prawdopodobieństwem zbliżonym do 25%.
